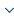1. Introduction
The D type knot is one of the core components of the bundling machine [1]. The wear of the cam on the drive gear plate causes low binding rate, low reliability, and insufficientlifetime. The certifiable bundling rate of D type knots made in Germany and the USA is 99% during their designed life, even though the wear rate of cams on drive gear plates is also relatively high [2-3]. At present, how to improve the service life of D type knotters has become the bottleneck in reducing the price of baling machines. Therefore, aiming at addressing the wear problem of the Cams of driving gear plates, this paper analyzed the knot failure and calculated its reliabilityso as to find the best maintenance strategy.The analysis is proven to be a service able process for the reliability estimation of D knotting machine.
2. D-Knotter Overview
A drive gear plate is an important part of the D type knotting machine. It provides power tothe whole knotting machine, whose quality affects the efficiency of the entire baler knotting. It comprisesone input shaft and three output lines for power transition. Output lines aredesigned to drive the other parts of the knot device movement by gear meshing and the cam mechanism. The outer rack has seven teeth, is evenly distributed on the axis of the coiling disc, and meshes with the incomplete gear mounted on the knot mouth, providing power output for the knotter jaw. The inner rack also has seven teeth, is a variant tooth that meshes with the incomplete gear mounted on the worm, and provides power output for the clip rope disc. The cam mechanism provides the power to diastasis the knot and cutrope for the off rope rod. There is a fixed phase difference between the two rack and the cam, and the phase difference determines the time sequence related to the cut rope and takes off the knot action after the knot mouth action [4-6]. The running time is short, fast, and highly precise, and the trajectory of the gear is also precise [7-9]. A new drive gear plate structure is shown in Figure 1.
Figure 1.
Figure 1.
D type knotter driver gear plate
Figure 2 shows the wear condition of a D type knotter drive gear plate working 20 thousand times without maintenance. Because of the contact between the off rope rod and the knotter jaw, the stress on the contact surface of the cam and the cam follower increases, causing serious wear and tear. Moreover, owing to no maintenance, the assembly accuracy of the cam and the follower decreases; as a result, displacement reliability will decrease, then transmission error increases, wear aggravates, and vicious circle finally occurs, resulting in D knot knotting failure. Therefore, this paper carried out the movement reliability analysis and established mathematical models for calculating the relationship between the wearof the cam and the reliability of the cam motion transmission.The analyzed lifetime of the D type knotter will be the originalinput knowledge for the optimal maintenance strategy.
Figure 2.
Figure 2.
The cam wear diagram on the drive gear plate
3. Failure Type and Failure Mechanism of Knotter
A knotter bears a variety of twisting, shear, cutting, pressing, and other complex forces, coupled with knotting in the field. Harsh working environments make damages of the knotter a frequent occurrence. Through the statistics of the working environment, the force, and the actual operation of the baler, five main failure modes are found: clamp rope failure, rope around failure, cut rope failure, upper claw failure, and rope buckle failure. Actual working condition through knotter shows that the drive gear, frame, casting off rope lever, knotting mouth, clamping rope mechanism, bevel gear, and cutting of the rope knife are the main factors that cause failures. The failure of the gear plate and the knotter frame are the most important factors for knotter failure.
3.1. Theoretical Analysis of Wear
Wear is a phenomenon of micro loss of surface material caused by friction. Wearis generally divided into abrasive wear, fatigue wear, adhesive wear, oxidation wear, fretting wear, and selective migration. The wear of the cam of the D type knotter is mainly caused by fatigue wear. This paper discusses the influence of cam wear amount on cam output motion parameters without considering the original error of the cam profile and deduces the mathematical relationship between wear volume and output motion parameters. Generally, wear can be divided into three stages: running-in stage, stable stage, and accelerated wear stage, as shown in Figure 3.
Figure 3.
Figure 3.
The curve of wear and tear path
Figure 4 shows the changing law of wear velocity. The crests on the friction surface formed in the manufacturing process are easily abraded in the running-in stage, which results in high initial wear speed, and the curve of wear velocity in this stage is concave. The wear rate tends to be constant after the crest is polished. After that the contact surface gap exceeds the allowable range, which causes a sharp increase in dynamic load, the wear rate and wear suddenly increase to form an accelerated wear and tear. Obviously, the normal work should be in the stable stage and only a specified amount of wear is allowed in the contact surface. If it is out of the specified range, the mechanism's motion reliability will be affected. The wear amount and wear rate are described mathematically as follows.
Figure 4.
Figure 4.
The curve of wear velocity changes
1) Running-in stage
Since the exact description of the wear curve is difficult and unrealistic, a similar curveis instead used in this paper. Thewear speed of the running-in stage varies from fast to slow, assuming the wear speed follows a uniform deceleration. The relationship between wear rate and time is modelled as Equation (1).
where ${{q}_{1}}$ is the wear rate at time $t$,${{q}_{0}}$ is the initial wear speed, and ${{\gamma }_{1}}$ is the changing rate of the speed, which is a random constant greater than zero.
The relationship between the wear amount $U$ and time $t$ can be obtained by integrating Equation(1), shown in Equation (2).
Equations (1) and (2) represent the functional relationship between wear amountand time in the stage of running-in.
2) Stable stage
The wear rate in the stable stage is a constant value, shown in Equation (3):
By integrating Equation(3),the relationship between wear amount and time in this stage can be reached, shown in Equation (4).
3) Accelerated wear stage
Wear speed is accelerated in this stage, and the relationship between the wear speed and the time can be found in Equation (5).
where ${{q}_{3}}$ is the wear rate at time$t$,${{q}_{0}}$ is the initial wear speed, and ${{\gamma }_{2}}$ is the changingrate of speed and a constant greater than zero.
The relationship between the wear amount $U$ and time $t$ can be obtained by integrating Equation(5), shown in Equation (6).
Equations (5) and (6) represent the functional relationship between wear amount and time in the stage of accelerated wear.
3.2. The Motion Analysis of the Cam Mechanism on the Drive Gear Plate
The coordinate diagram of cam mechanism on drive gear plate is establishedin Figure 5. Mathematical expressions for cam follower displacements are modeled by polar form. The polar coordinates of the point M at the center of the cam follower is expressed in Equation (7).
Figure 5.
Figure 5.
The coordinate diagram of cam mechanism on drive gear plate
where $\rho$ is the radial function,$\psi$ is the radial angle,$\phi$ is the angle of cam rotation, $\theta$ is the angle between the linear $O{{P}_{0}}$ and the $\chi$ axis,$s$ is the output displacement of the cam follower,${{r}_{b}}$ is the outer arc radius of the cam,${{r}_{r}}$ is the radius of the cam follower, and $e$ is the axial hole radius of the camshaft[1,10].
The cam profile curve equation is calculated in Equation (8).
where $\phi$ is the angle of the cam rotation, and it is a function of time.
Combining Equations(7) and (8), we get the relationship between $s$ and $\rho$.
By combining Equations (7) and (9), a mathematical relationship between the output displacement of the cam follower, the cam profile parameters, and the basic parameters of the cam can be established as Equation(10).
where $\phi $ is a function of the time, and ${{r}_{0}}$ and $e$ are constant.
3.3. Establishing Reliability Calculation Model of Cam Motion Containing Wear
The cam mechanism is connected with the high side.Wear occurs mainly in the cam’s contour line. Wear affects the radial error only. Relationships between wear and cam profile error and that between wear and cam follower output error are established.
1) Establishing the relationship between wear and cam profile error.
The wear relation in three stages has been established in the previous study, shown in Equation (11).
At a certain angle, the total error of the CAM profile is equal to the sum of the contour error without wearing and the wear amount, which is modelled in Equation(12).
where $\Delta$ is thetotal error of cam profile,${{\Delta }^{\text{*}}}$ is The cam profile error without wear, and $U$ is the total amount of wear.
The total cam profile error for the three wear stages can be calculated by combining Equations(11) and (12).
Running-in stage:
Stable stage:
Accelerated wear stage:
According to the statistics, the mean parameters of mean and variance in the three stages are calculated in Equations(16)-(21).
The expected value of the total profile error of the cam changes with time in different wear stages.The longer the time, the greater the error mean and greater the influence on the output motion.Hence, the characteristic values of the profile error of the cam in the three wear stages are obtained.
2) Establishing the relationship between wear and output error of the cam follower:
Symbol $\text{*}$ represents the ideal value, and the error is expressed by $\Delta $. Based on this, Equation(22) is obtained from Equation(9). Equation(22) at the ideal value is extended by first-order Taylor series expansion, shown in Equation(23).
The mean and variance of the cam follower displacements are calculated in Equation(24) under the circumstance of mean ofare respectively ${{\mu }_{\rho }},{{\mu }_{{{r}_{0}}}},{{\mu }_{e}}$,and the standard deviations are${{\sigma }_{\rho }},{{\sigma }_{{{r}_{0}}}},{{\sigma }_{e}}$. Wear only affects the profile error of the cam, and the eigenvalues of the cam profile error in the three wear stages are shown in Equation(25).
where ${{\mu }_{{{q}_{0}}}}$ and ${{\mu }_{{{q}_{c}}}}$ are the mean values of the initial speed of wear,$\sigma _{{{q}_{c}}}^{2}$ and $\sigma _{{{q}_{0}}}^{2}$ are the variances of wear speed,${{\mu }_{\gamma }}$ is the mean of the rate of velocity change, and $\sigma _{\gamma }^{2}$ is the variance of the rate of velocity change.
${{\mu }_{p{{z}_{1}}}}$,${{\mu }_{p{{z}_{2}}}}$, ${{\mu }_{p{{z}_{3}}}}$, $\sigma _{p{{\sigma }_{1}}}^{2}$, $\sigma _{p{{\sigma }_{2}}}^{2}$,and $\sigma _{p{{\sigma }_{3}}}^{2}$ are used to considerthe wear of ${{\mu }_{p}}$ and $\sigma _{p}^{2}$, and then three wear stages of the cam displacement output parameter error relationships (shown in Equations(26)-(28)) can be obtained by Equation(24).
Running-in stage:
Stable stage:
Accelerated wear stage:
Wear affects the mean and variance of the output displacement error of the cam.The longer the time, the greater the influence will be. The characteristic value of the cam profile wear speed and the allowable limit error characteristic value of the cam follower by motion simulation of the cam wearis required for calculating the displacement reliability of the cam including wear.
3.4. Motion Simulation of Cam Wear
First, the finite element model of the drive gear plate of d-type knotter is established, and then it is imported into the ADAMS. A virtual experimental prototype for the cam and follower engagement is established, as shown in Figure 6.
Figure 6.
Figure 6.
Virtual experimental prototype of D type knotter
The boundary conditions and initial loads are defined according to the actual working parameters of the D type knotter. At the same time, the speed of the drive gear plate and the torque of the spindle are inputs, and then the motion simulation is carried out.
We extract the simulation results of two aspects in the process of simulation.On one hand, we analyze the D type knotter successful knot boundary conditions to obtain the cam follower displacement allowable range and limit values.After many simulation results, the cam-follower displacement allowable limit error characteristic value $({{\mu }_{0}},{{\sigma }_{0}})$ is obtained. On the other hand, we obtain the stress load spectrum of the cam target contact surface. After obtaining the stress load spectrum of the contact surface of the cam, the stress damage analysis of the cam is carried out by ANSYS software to obtain the displacement damage amount and the cycle life. Then, we compare the displacement damage amount with the limit allowable wear amount. If the displacement damage amount is less than the allowable limit of the cam wear, we modify the cam finite element model according to the amount of damage. Then, we proceed to the next cycle of motion simulation until the displacement damage is greater than or equal to the limit of the cam, and then we stop the simulation. Concrete realization of the process is shown in Figure 8. In this way, we can get the maximum movement times before the failure of the cam and the maximum working life by multiple motion simulation. According to the maximum displacement damage, we can obtain the wear speed, and then we can get the characteristic value $({{\mu }_{{{q}_{0}}}},{{\sigma }_{{{q}_{0}}}})$ of the cam profile wear speed according to the results of multiple simulation.
Figure 7 shows the stress change versus time for the target contact surface without wear of the cam profile when driving the gear plate for two cycles in an ADAMS virtual prototype.
Figure 7.
Figure 7.
Virtual prototype simulation flow chart of cam
As shown in Figure 8, the maximum stress that can be obtained in the measurement interface to the cam target contact surface occurs at 0.529s and 1.19s, which are the times when the off rope rodtakes off the knot. Meanwhile, due to the contact between the off rope rod and the knotter jaw, the stress between The cam and the cam follower contact surface suddenly increases (the maximum stress is up to 1179N). The maximum stress is loadedin a cyclic form to the target contact surface of the cam in ANSYS software. The cycle time of the applied load is the design life of the D type knotter. Then, according to the actual material properties and motion parameters of the cam, the static damage analysis of the cam is carried out, and the damage displacement and deformation cloud map of the cam is obtainedas shown in Figure 9.
Figure 8.
Figure 8.
Virtual prototype simulation flow chart of cam
Figure 9.
Figure 9.
Virtual prototype simulation flowchart of cam
It can be seen from Figure 9 that the maximum displacement damage of the cam contact surface is 0.304mm. The D type knotter will failwhen the displacement damage amount of the cam contact surface reaches 2.34mm. The stress of the cam contact surface increases to 6000N at that time. It is knownthat when the displacement wear amount of the cam contact surface reaches 2.34mm, the D type knotter cannot be successfully knotted.
After multiple simulations, theD type knotting machine can successfully knot to the unsuccessful knotting. The limit wear diagram of the cam contact surfaceis obtained as shown in Figure 10.
Figure 10.
Figure 10.
Limit displacement wear of cam contact surface
From the simulation results shown above, the displacement allowable limit error eigenvalue of the cam followeris obtained according to the probability formula $({{\mu }_{0}},{{\sigma }_{0}})$=(2.219, 0.09). According to the flowchart shown in Figure 7, after 20 cycles of simulation, the wear displacement results are obtained, shown in Table 1 and Figure 11.
Table 1. Simulation results of cam wear displacement
| Simulation time | Wear displacement | Simulation time | Wear displacement |
|---|---|---|---|
| 1 | 0.304 | 11 | 0.864 |
| 2 | 0.339 | 12 | 0.964 |
| 3 | 0.376 | 13 | 1.076 |
| 4 | 0.416 | 14 | 1.201 |
| 5 | 0.46 | 15 | 1.34 |
| 6 | 0.509 | 16 | 1.494 |
| 7 | 0.564 | 17 | 1.664 |
| 8 | 0.626 | 18 | 1.851 |
| 9 | 0.696 | 19 | 2.056 |
| 10 | 0.775 | 20 | 2.34 |
Figure 11.
Figure 11.
The curve of the wear displacement of the cam contact surface
Table 2. Simulation results of cam wear speed
| Simulation time | Wear speed | Simulation time | Wear speed |
|---|---|---|---|
| 1 | 0.0408 | 11 | 0.1041 |
| 2 | 0.0455 | 12 | 0.1161 |
| 3 | 0.0505 | 13 | 0.1295 |
| 4 | 0.0559 | 14 | 0.1446 |
| 5 | 0.0618 | 15 | 0.1614 |
| 6 | 0.0684 | 16 | 0.1801 |
| 7 | 0.0758 | 17 | 0.2008 |
| 8 | 0.0841 | 18 | 0.2236 |
| 9 | 0.0935 | 19 | 0.2487 |
| 10 | 0.0408 | 20 | 0.2763 |
Figure 12.
Figure 12.
The curve of the wear speed of the cam contact surface
According to the results shown above, the eigenvalues of the wear speed of the cam contact surface can be calculated according to the probability formula: $({{\mu }_{{{{}}_{0}}}},{{\sigma }_{{{q}_{0}}}})$= (0.134, 0.083).According to the simulation results and the actual size parameters and motion parameters of the cam, the reliability of cam follower displacement, speed, and acceleration can be calculated in the three wear stages when the running time reaches the design life.
3.5. Calculation of the Displacement Reliability of the Cam on the Drive Gear Plate in the Stable Stage
According to Equation10 and the mathematical model of the cam on the drive gear plate, Equations(29) and (30) are obvious.
Table 3. The basic parameters of the cam on the drive gear plate
| The push travel angle ${{\phi }_{1}}$: | 0~63.170 | The radius of the cam follower | 1.25cm |
|---|---|---|---|
| The return angle ${{\phi }_{2}}$: | 63.170~123.170 | CAM rotation speedn: | 90r/min |
| The distance of run in cam followerh: | 2cm | CAM angular velocityω: | 3πrad/s |
| The radius of camshafte: | 2.8cm | Design service lifet: | 40000 time |
| The Outer arc radius of camrb: | 4.2cm |
Substituting the cam parameters into Equations (29) and (30), the following results can be obtained:
$s=1.181,\text{ }\rho =6.495,\text{ }\frac{\text{d}s}{\text{d}\rho }=1.108,\text{ }\frac{\text{d}s}{\text{d}{{r}_{0}}}=-1.166,\text{ }\frac{\text{d}s}{\text{d}e}=0.121$
For the cam in the steady wear stage, according to Equation (27) and Equation (31) can be obtained:
The original profile error of the cam is not considered. Therefore,${{\mu }_{\rho }}$=${{\mu }_{{{r}_{0}}}}$=${{\mu }_{e}}$=0,${{\sigma }_{\rho }}$=${{\sigma }_{{{r}_{0}}}}$=${{\sigma }_{e}}$=0. The above data is substituted into Equation (31), and the following results are obtained:${{\mu }_{\text{s}}}=1.105\text{,}$$\sigma _{s}^{2}=0.468.$The displacement error data of cam wear obeys the normal distribution of $\mu =2.219.$ When the displacement error of the cam wear is greater than 2.219mm, the D type knotter cannot be successfully knotted.According to the normal distribution, the statistical formula is:
Substituting the data into Equation (32), conclusions that $\beta $= 0 and the reliabilityis 0.9463 are reached.When the average error of CAM wear is 0.87 mm, the reliability is 0.9909 and the CAM been run to an average of 2230 times, namely the design of the CAM is about half the service life.
4. Conclusions
The cam mechanism equipped in the drive gear plate of the D type knotter under normal working condition works at asteady wearstage after the analysis. Without considering the original error of the cam profile, the cam and follower of the contact surface erosion rate will reach 2.3 mm at the D type knot’s design life, and the cam follower displacement reliability is 0.9463.The Dtype knotter will be unable to successfully knot when the wear is larger than 2.3 mm. The conclusion that the displacement reliability of the cam follower is more than 99% requires the mean value of the displacement wear of the cam contact surface to be less than 0.87 mm after multiple simulation and calculation. Meanwhile, the average working time of the cam is about half of the designed service life. Therefore, it is recommended that the maintenance for the cam mechanism of the Dtype knotter should be takenafter 20, 000 bales to ensure that the reliability of the displacement of the cam follower is higher than 99% andthe D type knotter canbe successfully knotted.
Acknowledgements
This work is supported by the National Natural Science Foundation of China (No. 51365031) and the National Key Research and Development Project of China(No. 2016YFD0701700).
Reference
“Reconstruction and Motion Simulation of D-Type Knotting based on Reverse Engineering, ”
DOI:10.6041/j.issn.1000-1298.2014.12.017
URL
[Cited within: 1]

D-bale knotter is one of the key parts of square baler, which influences the efficiency and quality of baler. Based on reverse engineering, D-bale knotter was reconstituted and the accuracy of working surfaces was analyzed. The results showed that more than 90% domain for all the working surfaces can achieve the precise level above. Reconstruction parts of D-bale knotter were assembled and then simulated by using the software Solidworks. The angular velocity and displacement data of twine holder, bill hook, wiping arm, and driving dentate disc were obtained and the movement relationships of these parts were further analyzed. This study will provide basic data for the optimization of D-bale knotter.
“Rotational Swashplate Pulse Continuously Variable Transmission based on Helical Gear Axial Meshing Transmission, ”
DOI:10.3901/CJME.2012.06.1138
URL
[Cited within: 1]

AbstractThe current research on pulse continuously variable transmission(CVT) is mainly focused on reducing the pulse degree and making pulse degrees a constant value. Current research mainly confined to find out new design parameters by using the method of optimization, and reduce the pulse degree of pulse CVT and its range of variation. But the fact is that the reduction of the pulse degree is not significant. This article presents a new structure of mechanical pulse CVT he rotational swashplate pulse CVT with driven by helical gear axial meshing. This transmission is simple and compact in structure and low in pulsatile rate (it adopts 6 guide rods), and the pulsatile degree is irrelevant to the transmission ratio. Theoretically, pulsatile rate could be reduced to zero if appropriate curved surface of the swashplate is used. Compared with the connecting rod pulse CVT, the present structure uses helical gear mechanism as transmission part and it avoids unbalanced inertial force in the former model. This paper analyzes the principle of driving of this transmission, presents its mechanical structure, and discusses its motion characteristics. Experimental prototype of this type of CVT has been manufactured. Tests for the transmission efficiency(when the rotational speed of the output shaft is the maximum) and the angular velocity of the output shaft have been carried out, and data have been analyzed. The experimental results show that the speed of the output shaft for the experimental prototype is slightly lower than the theoretical value, and the transmission efficiency of the experimental prototype is about 70%. The pulse degree of the CVT discussed in this paper is less than the existing pulse CVT of other types, and it is irrelevant to the transmission ratio of the CVT. The research provides the new idea to the CVT study.
“Pro/Engineer Simulation and Action Function Analysis of D-Type Knotter, ”
“Study on the Spatial Parameters of the Knotting Machine of the Square Bundle Balere, ”
“Design and Experiment of D-Type Knots Knotters on Chinese Small Square Balers, ”
DOI:10.3901/CJME.2014.01.154
URL
[Cited within: 1]

Since the knotters on the Chinese rectangular balers are imported from outside of the country, Chinese knotters with independent intellectual property rights is far away from being closed.In order to harvest a large quantity of straw in a short period on the small-scale lands of China, basic requirements on the knotters are summarized.Mathematical model of the knotter is also determined uniquely.Furthermore, theф-type-knots knotter equipped on the Chinese square baler to form theфtype knots is designed.Knotting rate experiments of theф-type-knots knotter on the test bench and in the wheat/maize straws covered fields are carried out to check the knotting performances of the knotter.The parameters of the formed knots are also tested.The experiments results show that the knotting rate of theф-type-knots knotter reaches 100.0%on the test bench without straws, while reaches 99.6%in the wheat straws covered field and 100.0%in the maize straws covered field.The average maximum force in the knotting process is 194.7 N in the lab experiment.The length out of the knots formed in lab is 15.9%–20.6%lower than the knots formed in the field experiment.The breaking force of the knots formed in the field is 115.9%–167.2%higher than the knots formed in lab due to the higher preload and interactions with the compacted bales.Highly relevant relationships exist between the breaking force of the formed knots and the maximum force in the forming process of the knots in the lab experiment.The designed knotter breaks out the embarrassing situation of the domestic knotters which don’t have independent intellectual property rights, and promotes the development of Chinese knotter technology, and the mathematical model is helpful for designing new type of knotters.
“Construction and Optimization of the Drive Plate for D-Type Knotting Machine with Square Straw Baler, ”
“Wear Analysis and Improved Design of Cutting Rope Tripping Mechanism, ”
“Drive Tooth Plate Modal Analysis of D-Type Knotter, ”
“Measurement of the Space Angle of the Knotting Machine of a Baling Machine based on Reverse Engineering, ”
“Structure and Motion Analysis of D-Type Knotting Device, ”
“Research on CAD /CAM of Acceleration and Deceleration Cam Mechanism, ”
“Study on the Motion Law and Design Basis of a Knot Cutter to Cut the Rope Tripping Mechanism, ”
“Simulation and Timing Analysis of D-Type Knotter and Its Auxiliary Mechanism, ”